Hitler and Dragonball Z

Posted on September 30, 2008 by admin

I’ve been busy lately, haven’t had much time to post anything. Here is a quick post. I ran across this video the other day. It’s a clip from a German movie Downfall, a movie about the last few days of Hitler’s life. The scene is one where the chief officers of the Third Reich finally give Hitler the grave truth on the imminent fall of Berlin and the Reich instead of sugar-coating all the reports to him as they had been. It’s a very intense and grave scene, but there are several videos where people have added subtitles that are completely non-sequitur: this one is where Hitler learns about the production for the live-action Dragonball Z.

I don’t know why, but I couldn’t stop laughing. Maybe the thought that there really are people as passionate about DBZ as a dictator is about his power. There’s also a hilarious comment on the YouTube page: “For the first time in my life, I completely agree with Hitler.”

Posted in General | 1 Comment

Trip to Burnet, pt. 2

Posted on September 2, 2008 by admin

I mentioned in my last post that there was one other interesting thing that I would mention. Among the various artifacts in the museum was an old desk. Looking at it, it had this sign on the front:
mormon desk
Mormons? In Texas in the 1850’s? This was news to me, and I’m a 5th generation Mormon. There was a photograph also:
mormon mill
(click for larger version)
Fortunately, the desk also had a newspaper clipping on it that gave a brief explanation of what exactly happened. The print was too fine for me to take an effective picture of the article, but the account can be found elsewhere online. This site had a good explanation of what happened. For those familiar with Mormon history in general, you can skip down to a couple of paragraphs before the section “Enter Texas”. For those not willing to spend the time to read the whole article, Lyman Wight was one of the leaders (a member of the Quorum of the 12) of the Church of Jesus Christ of Latter-Day Saints (i.e. the Mormon church) during Joseph Smith’s time. After the martyrdom of Joseph Smith, the majority of the saints went with the leadership of Brigham Young, the president of the Quorum of the 12. There were several groups that did not acknowledge his leadership, however. The largest group felt that leadership should be passed onto Joseph Smith’s sons, that group later became the Reorganized Church of Jesus Christ of Latter-Day Saints (changed to the Community of Christ in 2001). Another group went with James Strang, and they had their own very interesting history culminating in Beaver Island, Michigan.

Anyway, Wight had been living a little seperated from the main body of the saints in Nauvoo, having headed up a community that ran a sawmill up in Minnesota to produce all the lumber used in building the temple in Nauvoo. According to Wight, the last thing Joseph Smith had asked him to do was to move the saints to a safe haven in Texas. So Wight took the families that he had been the leader over and took them down to Texas. They first ended up in present day Austin, but moved on after a few seasons. One part of the legacy they left behind in Austin is two prominent streets in Austin, Exposition and Far West, both retain names originally given by these Mormons. They next moved to Fredricksburg, where a community of recent German immigrants had established a town. The German immigrants welcomed Wight and his followers, as the Mormons built a sawmill there that was of great benefit to the community, and also the Mormons there taught them how to farm and such, since many of the immigrants were poor urban Germans that had not farming experience themselves.

Things were fine for a few years, but a flood destroyed the sawmill and broke the Mormons there financially. They ended up mortgaging all their property, and moving about 50 miles north to Burnet. There they again built a sawmill and were very industrious, but Wight didn’t seem able to manage the finances of the group very well, and were again in deep debt. They group moved one more time to a settlement named Zodiac. A few years later, Wight died and no leader emerged among the group to take his place. Some of the families returned to Missouri and joined the saints that had remained there under the leadership of Joseph Smith’s son. Others stayed there and slowly blended into the local community, no longer distinguishing themselves as Mormons. It would be almost a century until there was again a population of Mormons in the central Texas area.

Posted in General | 3 Comments

Trip to Burnet

Posted on August 29, 2008 by admin

Last Saturday Ryoko wanted to get out of the house and go somewhere. This is actually harder than it seems. It being August in Texas, it’s 95 to 100 degrees every day, the only exception being if it happens to rain. Ryoko has very sensitive skin and being out in the hot sun for more than just a few minutes causes her to break out into a rash that takes weeks to clear up using both topical and ingested medicines. So anything outdoors is pretty much out until October at the earliest. Indoors, well, there aren’t so many options. There are a few museums and such, but all the free ones we’ve been to multiple times and we’re a little bored of them. There is a place that is an indoor play area for children, but it’s pretty steep – $3.00 per child under 3, and $7.50 per child 3 and over. That’s $10.50 just to have an indoor place to play, way beyond our budget.

So I looked a bit on the internet and found a place not too far where we hadn’t gone before: Burnet, TX. It’s maybe a half-hour drive to the northwest, and it has a small museum where a fort had been there back during the 1850’s.

There are a lot of period buildings in the compound, but due to the weather we mostly stayed inside the main building and looked at the artifacts in there. It is a small quaint little museum, reminiscent of the kind of places your class might go on a school field trip when you were in kindergarten or 1st grade.
In front of museum
(click for larger image)

Still, there were many very interesting artifacts there, even if a lot of them really didn’t have much to do with the frontier period of central Texas. If I had to guess a theme for the inside of the museum, it was “This is everything donated by the community that’s over 100 years old that we thought might be interesting to display.” There were two old pianos, a Model T Ford that served as an ambulance in WWI, samples of old barbed wire, many Indian arrow heads, lots of period clothing, old typewriters, on old telephone switch board, a hand printing press, a loom, spinning wheels, old maps, etc. etc.

Very coincidentally, the day we visited happened to be the day that the local civil war re-enactment group was having their muster. We went outside for a few minutes to see what was up. There was an Indian tepee which didn’t really seem to fit, but Ryoko thought it was novel so we took a picture.
Tepee

Sure enough, there was a line of men with muskets marching:
marching line
(click for larger)

So this being Texas, they were a regiment for the Confederate army. I was curious why they didn’t have the trademark grey uniforms, but it turns out that during the war, the south was so strapped for cash that most soldiers didn’t even have uniforms. After the men gathered in some shade under a tree (remember it’s 95~100 degrees out, around 70% to 80% humidity), the captain (only distinguishable by his ownership of a sword) took one of his soldiers with him to give the onlookers a bit of explanation and a demonstration of the musket.
musket demonstration
(click for larger)
(I personally thought the captain looked a lot like Chris Cooper, but maybe that’s just me) The soldier demonstrated how to load and fire the musket. It was interesting to see the slight technological changes that the musket had gone through since revolutionary war times. They are:

1.) Pre-measured powder packets wrapped in paper. The soldier had a satchel on his side with 40 or so of these packets with equal number of of lead balls. Loading consisted of grabbing a powder packet, biting the tip off, pouring the powder in the muzzle, dropping in a ball, then ramming it down.
2.) Mercury fulminate percussion caps that allowed for reliable firing, even when wet. After loading the powder and ball, a percussion cap was placed below the pin, and then the gun was ready to fire once the hammer was cocked.

That was all I noticed. They still didn’t have rifling, breech-loading, or single-unit ammunition (i.e. the gunpowder and bullet pre-assembled in one unit, what we think of today as ammunition).

So we went back inside (Ryoko was getting uncomfortable, and there were several bees that were far too interested in us) where we looked at the exhibits some more. Then I noticed something that I’ll talk about in my next post.

Finally, here’s a picture of Karisa looking at some of the maps on the wall:
Karisa looking at the maps

Posted in General | 1 Comment

How to get 92 heads in a row, pt. 2

Posted on July 22, 2008 by admin

Two posts ago I showed how many coin flips it would take in order to have a 98% confidence of getting 92 heads in a row (à la Rosencratz and Guildenstern Are Dead). The answer turns out to be \(3.874\times 10^{28}\) coin flips, which if you tried to do by yourself, it would take 100 billion times longer than the current age of the universe. Since I mentioned that only Wowbagger, the Infinitely Prolonged could pull off such a stunt, I think it’s only fair that any other solution also be Adamsian, at least in practicality if nothing else.

My friend Spencer proposed a Dyson sphere to power a huge number of coin-flipping robots. I think he’s on the right track and I had similar thoughts, however my ideas are a bit larger in scale and less detailed. I won’t go into detail on what kind of Dyson Sphere is best or such, since even simplistic models are fraught with difficulties and instabilities (a nice page talking about Dyson Spheres and some simple analysis is here). Instead, let’s just say that we can build some kind of large Dyson network in order to capture a significant portion of the Sun’s energy. We’ll be conservative and say that after light capture, conversion to useful energy, and then maintenance, etc. we can use 10% of the Sun’s radiant energy to power an array of coin-flipping robots.

Spencer also mentioned the concern that once you have good enough robots, that coin flipping is no longer random: exactly precise robots flipping exactly precise coins in the exactly precise way will give the exact same result every time. That may be the case, but we’ll assume that the robots and coins are made imprecise enough that there will be enough random variance in between all the robots to make the system truly random and fair (this is in all reality probably impossible, but we’re in Adam’s universe so we’ll assume it can be done anyway).

Since the robots don’t have to do anything but flip coins and report the outcome, we’ll say each robot consumes about as much power as a toaster oven, or 1000 W. The sun’s luminosity is \(3.846\times 10^{23} W\), so assuming we can use 10% of the sun’s energy we have:
\(\displaystyle{(0.1)\left (3.846\times 10^{26} W \right)\left (\frac {1\;\text {robot}} {1000\;W} \right) = 3.846\times 10^{22}\;\text {robots}}\)
This many robots would give us the same number of flips every second, so that will give us the required number of flips in:
\(\displaystyle{\frac{\displaystyle{3.874\times 10^{28}\text{flips}}}{\displaystyle{3.846\times 10^{22}}\textstyle{\frac{\text{flips}}{s}}}=1.007\times 10^6s\approx 11\; \text{days and}\;14\; \text{hours}}\).
Now that is a considerable improvement.

This potential solution does have some problems though, the most obvious being whether there is enough useful material in the entire solar system to build \(3.846\times 10^{22}\) robots, plus the Dyson power grid to run the whole thing, plus a maintenance system to keep it all in good working order, etc. If we limit ourselves to just the easy to use material, like just the asteroid belt, that limits us to about \(\small{2\times 10^{21}}\) kg of mass. Assuming a total of 10 kg for each robot (including Dyson network power generation, infrastructure, maintenance, etc.), that limits us to just \(2\times 10^{20}\) robots. This number of coin-flipping robots would then take 6.14 years to get the required \(\approx 3.874\times 10^{28}\) coin flips, which still isn’t bad at all. It might take several millenia to build the coin-flipping robot Dyson network, but once it was up and running you’d have your 92 heads in a row in just a few short years!

So lets say we let our coin flipping Dyson array keep running, say, until the Sun becomes a red giant in about 5 billion years, destroying our Dyson array. We would have

\(\displaystyle{\begin{array}{c}\left( 2\times 10^{20}\text{robots}\right) \left( \frac{\displaystyle { 1\;\text{flip}}}{\displaystyle { \text{robot}\;s}}\right) \left( \frac{\displaystyle { 3.1557\times 10^{7}\; s}}{\displaystyle {1\; \text{yr}}} \right) \left( 5\times 10^{9}\; \text{yr} \right) \\ \approx 3.2 \times 10^{32} \text{coin flips} \end{array}}\)

From this can we calculate how many coin heads in a row we can expect to get during this time? Our initial equation is
\(\displaystyle{F = 1-\left( 1-2^{-n}\right)^{0.5\,f}}\)
where F the confidence probability we we desire (we’ve been using 0.98, or 98%), n is the number of heads in a row, and f is the number of coin flips. Rearranging this for n we have:
\(\displaystyle{n = \frac{1}{\text{ln}\,2}\: \text{ln} \left( \frac{-0.5\,f}{\text{ln}(1-F)} \right)}\)

For some various confidence probabilities we have these results:

\( \begin{array}{ l l } \textbf{F} & \textbf{n} \\ 0.9999 & 120 \\ 0.999 & 120 \\ 0.99 & 121 \\ 0.9 & 122 \\ 0.75 & 123 \\ 0.5 & 124 \end{array} \)

So what does this mean? You have a 99.99% chance of getting at least 120 heads in a row, pretty much guaranteed. However, you only have a 50% chance of getting up to 124 heads in a row. What gives? We go from flipping coins for 6 years to 5 billion years, and the only improvement we get is an additional 28 heads in a row? That’s because each additional head in a row has half the probability of occuring, so the probability decreases exponentially with a linear increase in number of heads required. Conversely, for an exponential increase in the number of coin flips, we see only a modest linear increase in number of expected heads in a row.

Posted in General, Science | 5 Comments

Strange Meats

Posted on July 20, 2008 by admin

Last Wednesday my family and I returned to Austin from our (so far) annual trip to Japan to see Ryoko’s family. Since Ryoko’s mother passed away last year, this year is one of the important anniversaries of her death where a special memorial service needs to be held. So we went to Ryoko’s home for two weeks to attend the ceremony and then to spend some time with her family.

The afternoon after the ceremony (held in the morning), all of Ryoko’s family was gathered together and chatting, and the subject turned to exotic foods. When asked for my two cents, I said that I’m always willing to try something at least once, and that I like new culinary experiences. Then Ryoko’s cousin Akihiro chimed in: “I know a place not far from here where you can eat deer meat and wild boar! I’ll take you there this evening!

So that’s how I ended up going here to eat:
wild boar restaurant!
That’s Ryoko’s father and older sister about to go inside. It’s a tiny little place that I can most easily describe as ‘seedy’. The outside looks a little run-down, and inside a little more so. Here’s the front of the restaurant:
front sign
Basically the large white sign says, “All natural: Wild boar stew, game fowl dishes, wild deer dishes.”
I didn’t bother taking any pictures of the interior of the restaurant, but it isn’t hard to describe: low light and dingy, old faded posters of actresses and Enka (basically, Japanese country music) stars. There was even a calendar with a nude woman on it hanging on the wall. (I took it down, rolled it up and set it behind the old dusty karaoke machine when the cook was back in the kitchen. He never noticed.)

The first dish was some wild fowl fried with some onions. I didn’t even take a picture of it because at the time I didn’t realize it was anything but ordinary chicken. Neither the taste nor texture disabused me of that notion. It was pretty good though.

The second dish was grilled deer meat with onions:
deer meat
It had a slightly gamy flavor to it, but it was pretty heavily salted and peppered so it didn’t stand out much. It wasn’t very tough, and I thought it was quite tasty!

The third dish was none other than roasted wild boar with salad:
wild boar!!!!!
This meat was really, really tough. It was hard to chew, and there was tons of fat on every cut. The flavor wasn’t too bad though. Sort of a cross between pork and something really really gamy. I don’t mind gamy flavor so once I could chew it until it was soft enough I had no trouble eating it, but someone without a high tolerance for gamy tastes might have trouble getting it down.

Overall it wasn’t too bad, but the atmosphere definitely left a lot to be desired.

Posted in Personal | 3 Comments

How to get 92 heads in a row

Posted on July 19, 2008 by admin

A couple of posts ago I talked about how laughably improbable it would be to get 92 heads in a row on a fair coin. To sum up, the probability is:

\(\displaystyle\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\cdots\left[\text{92 times}\right]\cdots\left(\frac{1}{2}\right)=2^{-92}=2.017\times 10^{-28}\)

The probability of this happening is so abysmally low that you could flip coins your entire life and never expect to see this happen. Or could you? How many times would you need to flip a coin to see a reasonable chance of this happening?

A pointless question? Most certainly. But trying to answer pointless questions that can be solved by math is one of the trademarks of a geek. So a quick review of probability and statistics lead me to the Geometric Distribution, which gives the probability of an event occurring after a given number of trials.

\(\displaystyle{P=p\left( 1-p\right)^{k-1}}\)

Here p is the probability of the event occurring once in one trial, i.e. \(2.017\times 10^{-28}\). k is the number of trials, and P is the probability of the event occurring once within k trials. However, this equation doesn’t quite give us the probability distribution we need. This function will give us the probability of getting exactly one event (heads 92 times in a row) out of k trials (flipping a coin 92 times in a row k times). We’re not interested in the probability of exactly one success, we’re interested in the probability of one or more successes. For that, we need the related Cumulative Distribution Function. Basically it’s the sum of the probabilities of 1, 2, 3,… up to k successes out of k trials. It’s actually pretty easy to derive without performing sums for arbitrarily large values of k. Since we want one or more successes, that means the only thing we don’t want is a failure for every trial. The probability of a single failure is simply \(1-p\), so the probability of k failures is \(\left( 1-p\right)^{k}\). Since we want every possible combination except every trial a failure, we just subtract this from 1 (the sum of all possible combinations is of course equal to one). This function comes out to be:
\(\displaystyle{F=1- \left( 1-p \right)^k}\)

Choosing a reasonable number for F, we’ll select 0.98. Solving the above equation for k we get:
\(\displaystyle{k=\frac{\text{ln}\left( 1-F\right)}{\text{ln}\left( 1-p\right)}}\)

This equation is exact, but it has a big problem. No normal calculator or computer is going to be able to calculate the answer because of the denominator. The logarithm of one is zero, so the logarithm of a number very very close to one is a very very small number. But no normal calculator is going to be able to handle \(\text{ln}\left( 1-2^{-92}\right)\approx\text{ln}\left( 0.999999999999999999999999999798\right)\)
(Note I said no normal calculator. I used Mathematica for this and it works fine. But anything limited to double-precision arithmetic isn’t going to get you there.) Fortunately there is a convenient Taylor series expansion for \(\text{ln}\left( 1-x\right)\). It is

\(\displaystyle{\text{ln}\left( 1-x\right)=\sum_{i=1}^{\infty}\frac{x^i}{i}}\)

The first term in the series will give more than sufficient precision in this case, so we have
\(\displaystyle{k=-\frac{\text{ln}\left( 1-F\right)}{p}}\)

This tells us how many trials we we will need to to have a 98% confidence of getting 92 heads in a row, but it doesn’t tell us how many coin flips we will need. Now a trial is defined as 92 coin flips, and if all 92 are heads it is a success, otherwise it is a failure. However, we don’t need to do all 92 flips each time, as soon as we get our first tails, we already know that the trial is a failure and we can start over. Since a fair coin is going to result in tails half of the time, then that means on average we will have two flips for every trial. So if f is the total number of coin flips we will need to get a 98% confidence, then:
\(\displaystyle{f=-\frac{2\;\text{ln}\left( 1-0.98\right)}{2^{-92}}=3.874\times 10^{28}}\)

This is a very very big number. If we flipped a coin once a second, how long would it take us to get this number of flips?
\(\displaystyle{3.874\times 10^{28}\,\text{flips}\left( \frac{1\;\text{s}}{1\;\text{flip}}\right)\left( \frac{1\;\text{yr}}{3.1557\times 10^{7}\;\text{s}}\right)=1.22\times 10^{21}\;\text{yr}}\)

And how long is this? This is really, really long. Astronomers estimate the current age of the universe to be \(1.373\times 10^{10}\) years old. That puts it as 100 billion times longer than the current age of the universe. This is a feat that could only be pulled off by, say, Wowbagger, the Infinitely Prolonged. According to this fascinating article on the eventual heat death of the universe, at this point there will be no matter left in the universe but white and black dwarfs (and black holes, but I don’t know if they’re considered to be matter within our universe or not), and they will be flung from their orbits due to gravitational radiation.

So is all lost? Is there no way to get 92 heads in a row? For all practical purposes, yes, there is no way. As for impractical purposes though, in a subsequent post I’ll detail a way that we could accomplish it long before the death of the universe using a scheme that in all reality would only make sense in a Douglas Adams’ universe.

Posted in Science | 3 Comments

The worst of 80’s sci-fi (with some 90’s thrown in)

Posted on June 20, 2008 by admin

A few months ago on Sakeriver there was a discussion about the best SF TV series. I submitted the following which I’m reproducing here because I’m lazy:

Talking about the best SF series is fun and all, but I personally much more enjoy talking about the worst of SF (we’ll limit ourselves to TV series for now). I’m sure that well-known turds like Voyager or Excalibur will be mentioned, but due to nostalgia I’m somewhat partial to 80’s TV shows. Here are some examples:

Manimal
Just the name of this show alone is distateful…

The Invisible Woman
I think it was a TV special and not a full series, so it may not technically qualify.

Misfits of Science
Notable for including Courtney Cox long before her Friends fame.

The Ghost Busters
Not what you’re thinking. This is long before the movie Ghostbusters. This was a children’s live-action show in 75-76. Columbia actually licensed the name from Filmation to make the movie. The movie was so popular that Filmation then came back and tried to cash in with the horrible cartoon Ghostbusters (some of you may remember this one). Columbia was none too happy about that since they had made the franchise a hit, so they hit back with The Real Ghostbusters, which is probably the one you think of when you think of a Ghostbusters cartoon. However this one sucked too, since the actors in the movie raised a lot of stink about their likenesses in the TV show, so they ended up being drawn differently, Lorenzo Music (who also did the voice of Garfield) got replaced with Dave Coulier for Peter Vankeman’s voice, etc. Slimer was changed from a gluttonous villian to an extremelly annoying slapstick sidekick, and then later usurped the actual main characters not unlike how Fonzie usurped Happy Days, Urkel would usurp Family Matters, and Elmo would usurp all of Sesame Street a decade later. But I digress.

Small Wonder
Ugh. I don’t think I need to say much about this show.

Not Quite Human (go to 3:20, this is all I could find)
Coasting off of his (relative) success from The Boy Who Could Fly, Jay Underwood starred in this made-for TV movie back in 1987. Co-starring a phoned-in performance by Alan Thicke as Chip’s father, he must have needed an extra paycheck in between seasons of Growing Pains or something. They actually made a couple of sequels for this, Not Quite Human II and Still Not Quite Human.

Out of This World
Not to be confused with the video game that had no relation, this was a crappy Saturday afternoon sitcom cut from the same mold as Small Wonder (and started around the same time, it ran from 87-91). The girl has a human mother and alien father, and her father grants her the ability to stop time at will. Instead of doing the logical thing anyone with this power would do (i.e. steal anything you want, take over the world, be a totall bad-*ss, etc.), she generally used it just to get out of stupid farcical situations that seemed straight out of Saved by the Bell.

No list of horrible Sci-Fi shows would be complete without some Super Sentai series and their derivatives. Instead of focusing on the well-known Power Rangers (which are certainly worthy of inclusion on this list), I’d like to introduce some of the less well-known copycats and spinoffs.

VR Troopers
This show, like Power Rangers, was produced by taking the action suit scenes from a Japanese Super Sentai show and re-shooting all the other scenes with new actors. What made this different though, is that they actually combined three different Japanese shows: Super Machine Man Metalder, Dimensional Warrior Spielban, and Space Sheriff Shaider. This of course produced a convoluted and unintentionally hilarious plot (Fans of Robotech and Voltron are knowingly nodding their heads here).

Superhuman Samurai Syber-Squad
Yes, that really is the name of the show. Another Tokusatsu adaptation like Power Rangers and VR Troopers (and Big Bad Beetle Borgs, but I don’t even want to mention them…), this one actually had lower production values than the others, if you can believe it. Notable for the supporting cast role of Troy Slaten who would later go on to play Jerry Steiner in Parker Lewis Can’t Lose.

My favorite of all though, has to be…
Tattooed Teenage Alien Fighters From Beverly Hills
Instead of licensing inexpensive footage from a post-run Japanese TV show, this show was actually 100% original, as far as it wasn’t a complete rip-off of Power Rangers and its derivatives. But as you might surmise by the name of the show alone, it was really, really bad. How it survived for 40 episodes I’ll never figure out, because my brother and I would laugh our way through Superhuman Samurai Syber-Squad because it was so horrible, but TTAFFBH wasn’t even very watchable to make fun of. I do remember one episode where Zsa Zsa Gabor had a cameo, though (the heroes were sent to an alternate universe where Zsa Zsa had just been elected Governor of California or something to that effect). There was another humorous episode where they broke the monster-a-day format. In this episode, the big evil boss left for a while and left his lieutenant in charge. The lieutenant, wanting to prove himself by defeating the heroes, keeps on sending monster after monster after monster instead of just giving up for the day after the first one is defeated. The heroes get overwhelmed and are about to be defeated when the big evil boss comes back, recalls the monsters, and berates his lieutenant for not ‘doing it correctly’ by not following the monster-a-day formula. Years later it reminded me of Dr. Evil talking with his son Scott in Austin Powers about being defeated because of following standard bad-guy clichés.

Anyone else got some really bad SF TV shows, preferably with videos so that all can enjoy in the campy badness?

Posted in Entertainment | 3 Comments

People are predictably irrational in actions involving uncertainty

Posted on June 15, 2008 by admin

I ran across this page that is an outline for a lecture given by a professor of statistics at Berkeley. The title of his talk is “The top ten things that math probability says about the real world”, but he just glosses over six of them and spends the majority of his lecture discussing the last four. Still, all of the points are valid and important, in fact a lot of his lecture covers subjects that are pet peeves of mine. But the one that never ceases to amaze me is is the title of my post: people are predictably irrational in actions involving uncertainty.

Take for example the opening scene in Rosencrantz and Guildenstern Are Dead, where they are flipping the coin. Rosencratz (or is it Guildenstern?) gets heads something like 92 times in a row. Now assuming a fair coin, the odds of that are laughably improbable: \(2^{-92}=2.019\times 10^{-28}\) . You’d have a millions of times better chance of winning the lottery than achieving this feat. In fact, given a lottery that has a one in one billion chance of winning, you’d have a better chance of winning said lottery 3 times in a row then you would of getting 92 heads in a row on a fair coin.

Proof: \(\left(1\times 10^{-9}\right)^3=1\times 10^{-27}>2.019\times 10^{-28}\).

But that’s not what’s important here. The issue in question is what people will predict the next coin flip to be. If they see the large number of successive identical coin flips, and you then ask them what the probability of the next flip also being heads is, they will usually give one of two answers: 1) It is most likely to be heads, because the coin is obviously ‘on a roll’ of heads. 2) It is most likely to be tails, because it’s had so many heads in a row that there is a ‘negative balance’ of tails that needs to be met. This is despite any and all assurances that the coin is perfectly fair. So the real answer is of course, 0.5 probability of heads, and 0.5 probability of tails. This is always true, no matter what the previous record of instances may be. The thing that many people fail to realize is this:

In any simple game of pure chance, every turn/round/instance is completely independent of previous turns, and and every single turn has the exact same probability every time. This is how casinos make the majority of their money.

So why are most people so predictably irrational in such situations? Obviously I’m not a psychologist (or other such similar profession, but see this slide from the end of the lecture), but I think it has to do with the fact that as humans, we almost never have to make judgments in situations where the outcome is truly random. Such situations have only arisen quite recently in human history with the advent of gambling. And even then there is only a subset of gambling games that are purely random (like craps or roulette, assuming they are truly fair) while many have a combination of chance and skill (card games fall into this category) and some are flat out not fair (slot machines).

In most everything that we deal with in daily life, even when there are events that seem random when we we observe them, they are almost never random. For example, take my daily bus commute. Even though the bus has a regularly scheduled time to arrive, from my perspective it appears random within a time frame of +/- 10 minutes. Also how long it take to arrive at school or home also appears to be random, with a total time of anywhere from 15 to 45 minutes, depending on traffic. But in reality, both when the bus comes and how long it takes to arrive at my destination are not random at all. The problem is that the number of variables that go into determining these two times are so vast and unpredictable that the end result may as well seem to be random when it isn’t.

Back to my former point though, I think we as humans tend to find pattern and correlation in many things (even when they don’t exist) because finding correlations and patterns is extremely useful. Such thought processes have fueled man’s scientific progress, and help humans navigate the dangerous minefield of social interaction. It has its downsides though. People losing lots of money in gambling is obvious, but also things like finding pictures of Mary or Jesus in just about anything, or the existence of most every pseudoscience out there (numerology, cryptozoology, paranormal phenomena, etc.).

Posted in Science | 4 Comments

Documents revealed about Taishō Emperor

Posted on June 5, 2008 by admin

My wife pointed out a very interesting article to me this morning about the Taishō Emperor, who was the emperor of Japan from 1912 to 1926. Specifically, the Imperial Household Agency released some of the official records from his reign. What makes the releasing of the documents news-worthy is that it is the first time the Imperial Household has ever admitted the mental and physical deficiencies of the Taishō Emperor. I couldn’t find the article at all anywhere in English (no surprise, the various Japanese news outlets seem to be very selective about what news they release to the ‘rest’ of the world), so I’ll give a quick translation.

大正天皇　「実録」３度目公開　病状の深刻化記す。
Newly released documents reveal health problems of the Taishō Emperor.

宮内庁は４日、大正天皇の動静を記録した「大正天皇実録」の一部（複製本）を公開した。０２、０３年に続く公開で、１９２１（大正１０）年７月から、死去して多摩陵に埋葬される２７（昭和２）年２月までが対象。深刻化する病状のほか、２１年に摂政となった裕仁皇太子（昭和天皇）の動静などが記されている。
On the 4th of this month, the Imperial Household Agency released reproductions of official Imperial records from the Taishō Emperor that contained details about the Taishō Emperor’s health. The released records cover a period of 2~3 years from July of 1921 until the burial of the Emperor at Tamaryo. In addition to the Emperor’s worsening condition, the documents also revealed that due to those conditions in 1921 Crown Prince Hirohito (later Showa Emperor) was placed as Regent on the Imperial throne.

実録は全８５冊で、今回は巻７７から巻８５の計９冊が公開された。宮内庁によると、「個人情報」を理由に塗りつぶした部分は、今回公開した全約１６万字のうち２％程度。これで即位から死去までが公開され、未公開は生まれてから即位までの４７冊となる。
The complete records contain a total of 85 volumes, of which the 9 volumes from number 77 to 85 have been released. According to the Agency, of the 160,000 characters contained in the released records, about 2% have been removed because it contained ‘personal information’. Including those just released, now all the records from the Taishō Emperor’s enthronement until his death have been released, leaving 47 more volumes covering the Emperor’s birth until his enthronement that have yet to be released.

今回公開分によると、２１年１１月２５日の裕仁皇太子の摂政就任時には「大正三年頃ヨリ軽度ノ御発語御障害アリ、其ノ後ニ至リ御姿勢前方ヘ屈セラルル御傾向アリ」「殊ニ御記憶力ハ御衰退アリ」などと病状について記されている。２３年９月１日の関東大震災の際には大正天皇は栃木県日光市にいたが、被災者のため１０００万円を寄贈したとの記述もある。
The released documents contain the following statements that detail the Emperor’s health problems around the time that Crown Prince Hirohito was inaugurated as the regent (note from Derek: these direct quotations are really hard for me to translate, because of the archaic style used in the official record, sorry if they’re not up to snuff): “His majesty’s speech impediment has become slightly worse since the the 3rd year of his reign (1915)、and recently his posture has shifted forward with a tendency to lean over.” “His majesty is developing pronounced memory problems.” The volumes also contain an account of the Taishō Emperor donating 10 million yen to victims of the Great Kanto Earthquake of 1923, when the Emperor was residing in the city of Nikko in Tochigi prefecture at the time.

実録は、天皇の動静や政治、外交上の重要事項などを記した文書。大正天皇については、死去後の２７年７月に当時の宮内省が編さんに着手し、３７（同１２）年１２月に完成した。
The released volumes contain accounts of the Emperor’s condition and important political and diplomatic matters during the Emperor’s reign. The Imperial Household Department began compiling the official records in 1927 after the death of the Taishō Emperor, and were completed in December of 1937.

Continue reading →

Posted in General | 1 Comment

This Sailor Moon thing is a little wierd

Posted on May 23, 2008 by admin

Since we speak mostly Japanese at home, most of the children’s books we have for our girls are also in Japanese. Recently we received some older children’s books from another Japanese family whose children are older and have grown out of them. Among them were 4 Sailor Moon storybooks. For those who haven’t heard of it and can’t be bothered to look it up, it’s essentially an all-girls Power Rangers kind of story. My girls currently love watching episodes of Pretty Cure 5 which is pretty similar, so I figured they would like Sailor Moon too.

So the other night I was reading them some books before bedtime, and Karisa asked me to read one of the Sailor Moon books we had just got. I thought the whole experience was so strange that I scanned the whole thing and put it here. So here we go!
(Note: you can click on the small images to see the full-sized ones. They are really big, but with the readership of this blog the bandwidth shouldn’t be a problem.)
I’ll do a simple text translation in italics, and then my comments in normal text.

SM01
Episode 27 of Sailor Moon S (Super): Haruka and Michiru are also Sailor Scouts!

SM02
Usagi (Sailor Moon’s secret identity) and her friend Minako (Sailor Venus) run into their friends Haruka and Michiru outside of the gym. However a suspicious woman in the car has followed them! “They must have the Talismans I’m looking for!”

(Upper-left corner) The bad guy, Unknown Professor, tells his underling Yujial, “get the Talismans, and the world will be ours!”

OK. the only thing that really wierds me out here is the girl in the far left, Haruka. She looks more like a pimp then she does a girl, and in fact you can’t tell she’s a girl at all until she transforms later. I also like how the mysterious bad-guy is a professor. Way to enforce negative stereotypes concerning academic achievement to young girls. Sailor moon is hardly the first to do this though, in Gatchaman (Battle of the Planets in English) the ultimate bad guy was Professor X.

SM03
Yujial chases them into the gym, where they are trapped in the locker room. First she attacks Minako. “Your spirit is mine!” (shoots her with purple light syringe-thing). They try and run away, but are trapped by Yujial and her ally, Diamond Door-nobu-da!

OK, this Door-nobu-da has to be the stupidest monster I have ever seen in any sentai series, and I include all of the original Power Rangers in that statement. She has a doorknob sticking out of her forehead, two more wrapped doorknobs for hair buns, a locker door attached to her left hand, and for a right hand she has what looks like a giant crappy Swiss army knife.

SM04
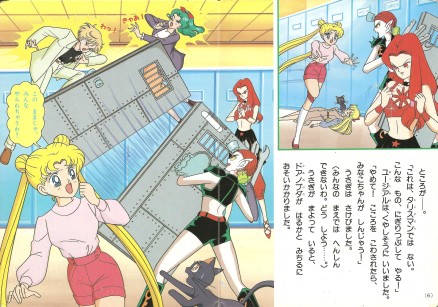
Yujial realizes that Minako’s spirit isn’t the Talisman she’s looking for. “I’ll destroy this worthless thing.” “No, don’t! She’ll die!” Yells Usagi. What can Usagi do? She can’t transform into Sailor Moon in front of her friends, they’ll find out who she is! While Usagi hesitates, Door-nobu-da attacks Haruka and Michiru!

So Door-nobu-da’s attack consists of… shooting doors at people.

SM05
Usagi has no choice. She transforms into Sailor Moon! Haruka and Michiru are suprised. “Usagi is Sailor Moon??!!” Usagi uses her special attack to return Minako’s spirit. “Moon Spiral Heart Attack!”

If you think that special attack has a wierd name, just wait.

SM06
“Destroy these girls!” Yujial commands. Door-nobu-da shoots door after door at Usagi, knocking her down. Haruka and Michiru come to her rescue. They are actually Sailor Scouts too! Haruka transforms with the words “Uranus Planet Power Makeup!” Michiru transforms with the words “Neptune Planet Power Makeup!”

OK, this has to be coincidence, but it just seems weird. The somewhat gender-ambiguous Haruka is… Sailor Uranus. I’ll just leave it at that. Also, take a look at the weapon that Yujial has pulled out. It looks like a chopped vacuum cleaner (complete with motorcycle handlebar on the bottom) and says ‘Turning 360 degrees’ on that half, and then connected with some hoses to another cylinder with a picture of an ear of corn on it. I have no idea what to make of this.

SM07
Usagi is shocked to find out that Haruka and Michiru are secretly Sailor Scouts. The duo then unleash their special attacks against Door-nobu-da, “Deep Sub-maji!” “World Shaking!” Door-nobu-da is destroyed! Then Yujial attacks. “I’ll show you my power! Fire Buster!”

OK, I have no idea what Sailor Neptune’s attack here is supposed to be. It’s written ディープ　サブマージ (diipu sabumaaji), but I have no idea what it’s supposed to be in English, assuming it comes from some English word in the first place.

SM08
When Yujial is about to finish the two off, Minako comes to the rescue! “Venus Star Power Makeup!” She then attacks Yujial. “Venus Love-me Chain!” Now surrounded by four sailor scouts, Yujial runs away. “I’ll get you next time!”

‘Venus Love-me Chain’ doesn’t seem like a name for a very potent finishing move, but I guess if it works you can name it whatever you like.

SM09
“Usagi is overjoyed to find out that her two friends are also Sailor Scouts. “Let’s fight together from now on!” she says. However Uranus and Neptune refuse. “Our mission is to find the Talisman. That has nothing to do with you two.” And before Usagi and Minako can answer, Haruka and Michiru leave. “Why can’t we be friends?” Minako asks. “I don’t know, but I think we’ll see them again.”

The mysterious allies disappear! Will we ever see them again? As long as the ratings hold, you better believe it!

SM10
“Hey girls! Get your parents to buy all these other Sailor Moon books!”

Posted in General | 11 Comments